The Psychophysics of Loudness
Review of Basic Concepts
Our ears are not at all interested in the total acoustical energy which reaches the eardrum. They are, however, sensitive to the rate at which the energy arrives i.e. the acoustical power (in Watts). This rate is what determines loudness.
Intensity, Sound Level, and Loudness
The amplitude of the eardrum oscillations leads to the sensation of loudness. This amplitude is directly related to the average pressure variation Ip, of the incoming sound wave and hence to the acoustical energy flow or intensity I reaching the ear.
There are two limits of sensitivity to a tone at a given frequency:
- A lower limit - the threshold of hearing 10
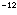Watt/m
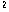= 0 dB, and - An upper limit - the threshold of pain 1 Watt/m 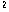= 120 dB.
These two limits vary from individual to individual and depend on the particular frequency under consideration. In general for a tone of 1 KHz the interval between limits is largest.
Because of the tremendous range, from 1 watt/m to 10 watt/m the unit of watt/m is impractical. The JND of a given stimulus is usually a good physical "gauge" to take into account when it comes to choosing an appropriate unit for the physical magnitude.
Experiments show that the JND in tone intensity is roughly proportional to the intensity of the tone. The magnitude used - the decibel - accomplishes three simultaneous objectives:
- A compression of the whole audible intensity scale into a much smaller range of values,
- The use of relative values (for example relative to the threshold of hearing) rather than absolute ones, and
- The introduction of a more convenient unit whose value closely resembles the minimal perceptible change of sound intensity.
Firstly we adopt the threshold of hearing at 1 KHz of 10 Watt/m as our reference intensity 
. We can then calculate the relative quantity Sound Intensity Level SL
SL = 10 log(I/) (dB)
For the threshold of hearing I/ = 1 and SL = 0 dB.
For threshold of feeling I/ = 10
and SL = 10 x log 
12 = 120 dB.
Whenever the intensity I is multiplied by a factor of 10, one just adds 10 dB to the value of SL. When the intensity is multiplied by a 100, one must add 20 dB etc. Here are some useful relations:
| Change in SL | What happens to the Intensity | |
| +(-)1 dB | X(div.) by | 1.26 |
| +(-)3 dB | X(div.) by | 2 |
| +(-)10 dB | X(div.) by | 10 |
| +(-)20 dB | X(div.) by | 100 |
| +(-)60 dB | X(div.) by | 1000000 |
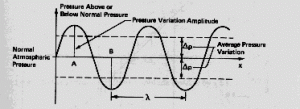
There is a relationship between the intensity of a sine sound wave and the value of the average pressure oscillation associated with the wave.
p = average pressure oscillation = pressure variation amplitude divided by 
, in Newton/m
V = velocity of the sound wave, and
= the air density.
For normal conditions of temperature and pressure I = 0.00234 x (p) (watt/m). Since according to this relation I is proportional to the square of Ip, we have
Hence, we can introduce the quality called sound pressure level:
Funny things seem to happen with the SPL when we superimpose two sounds of the same frequency and phase. According to the table above, doubling the intensity adds a mere 3 dB to the sound level of the original sound, whatever the actual value of the SL might have been. Superimposing ten equal tones in phase only increases the resulting SL by 10 dB.
To raise the SL of a given tone by 1 dB, we must multiply its intensity by 1.26 (or add a tone whose intensity is 0.26 that of the original).
The minimum change in SPL required to give a detectable change in the loudness sensation (JND in sound level) is roughly constant and of the order of 0.2 - 0.4 dB in the musically relevant range of pitch and loudness. Thus the unit of SPL or SL, the decibel, is thus reasonably close to the JND.
The perceived loudness of a sound, is however not directly proportional to its intensity. A built-in safety mechanism cuts down the sensitivity of the ear as intensity increases. When the intensity of a sound is doubled, the perceived loudness increases by only about 23%. Also, our perception of intensity varies with frequency - as we shall see later. Keeping frequency constant at say 1 KHz (1000 Hz),
| Intensity (watt/m) | Perceived Loudness | SL (dB) |
| 1 | Threshold of Feeling | 120 |
| 10-3 | Extremely loud (fff) | 90 |
| 10-4 | Very loud (ff) | 80 |
| 10-5 | loud (f) | 70 |
| 10-6 | Moderately loud (mf) | 60 |
| 10-7 | Soft (p) | 50 |
| 10-8 | Very soft (pp) | 40 |
| 10-9 | Extremely soft (ppp) | 30 |
| 10-12 | Threshold of Hearing | 0 |
N.B. : The intensity at the threshold of feeling is one trillion (10) times greater than the intensity at the threshold of hearing.
Intensity Masking
There is an alternative way of looking at the JND of intensity or sound level. Instead of asking how much the intensity of one given tone must be changed to give a JND effect, we ask:
What is the minimum intensity I2 that a second tone of the same frequency and phase must have to be noticed in the presence of the first one I1 whose intensity is kept constant? That minimum intensity I2 is called the threshold of masking. The original tone is called the masking tone and the additional tone is called the masked tone. Masking plays a key role in music.
The relation between the masking level ML (SL of tone threshold) and the JND of a sound level can be found using relation SL = 10log (I/) and taking into account the properties of logarithms. For e.g. for a JND of 0.2 db one obtains a ML that is 13 db less than the SL of the masking tone; a JND of 0.4 db corresponds to a ML that is 10 db below the masking tone.
Loudness
The psychological magnitude loudness is associated with a given SPL. Judgements of whether two sine tones sound equally loud show fairly low dispersion among different individuals. Judgements on "how much" louder one tone is than another require previous conditioning or training and yield results that fluctuate greatly from individual to individual and from occasion to occasion.
Curves of Equal Loudness
Tones of the same SPL but with different frequencies are in general judged as having different loudness. SPL is thus not a good measure of loudness, if we inter compare tones of different frequency. Experiments have been performed to establish curves of equal loudness, taking the SPL at 1 KHz as a reference quantity. These are shown below
Fletcher and Munson curves
pressure = 
or Force = difference in pressures times surface area F = (p-p')S
W = Fx = (mv)/2 . Unit is newtons/metre = Joule
W = work done, F = force, x = distance travelled , m = mass, v = velocity
The velocity of transverse elastic waves is given by:
where d = "linear density" of the medium i.e. mass per unit length (in kg/m) Thus, then more tense a string is faster the transverse wave will travel ( higher frequency). On the other hand, the more dense it is, the slower the waves will propagate.
For longitudinal waves the propagation speed in a medium of density (in kg/m3) and where the pressure is p (in Newton/m) is given by:
For an ideal gas the ratio p/ is proportional to the "absolute" temperature. Although air is not 100% ideal it behaves approximately so, and the velocity of sound waves may be expressed as
P = mechanical power. Units are Joule/sec called Watt
Roederer, Juan : Introduction to the Physics and Psychophysics of Music: The English Universities Press, London 1973.
Back to main course The Physics And Psychophysics Of Sound & Music
Back to The Psychophysics of Hearing